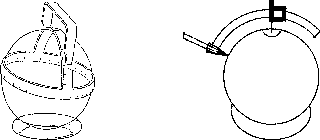From geometry to geometries:
an aspect of changing the geometry curriculum

Istvan Lenart
Postgraduate Medical School Central Research Group
H-1145 Amerikai ut 40/a Budapest
Hungary
100324.436@compuserve.com

Posing the problem

In the last four decades, curriculum changes have always been in the focus of interest of mathematics educators and teachers. Still, the problem is as open and acute as ever. The reasons are many, and the literature is vast, so I try to pick out some of the points which appear to be the most relevant from the point of view of the present paper.
One of the reasons for changing the curriculum is to influence and improve the public image of mathematics and mathematicians. At ICME-7, P. Ernest characterized the public image of mathematics as `cold, abstract, theoretical, ultra-rational, primarily masculine, largely remote and inaccessible to all but the most talented albeit important to society' (Cooney, 1994). I add that recognizing the importance of mathematics does not help ease mathematics anxiety. On the contrary, many people feel that their personal prosperity and success in life depend on a discipline which is unknown, incomprehensible, even hostile, for most of them. Accordingly, the image of mathematicians and teachers of mathematics can only too often be characterized with similar adjectives, sometimes also including terms like `condescending', even `conceited'. I cannot resist quoting a usual phrase of a mathematician of high renown, to his students: `Four dimensions are a trifle for me, but I can easily handle as many as five dimensions. A person who can only manage three dimensions is not worthy of the name `human being'! This image of the subject evokes symptoms such as the fear of success (Leder, 1980) that makes adolescent children prefer to deliberately avoid success in mathematics, for fear of losing the respect of their peers (Balacheff, 1990). Apparently, no breakthrough in this regard has taken place during the last four years.
Recently, a growing number of authors have analyzed the link between mathematics and politics, and the message of mathematics to the students regarding their own place and role in society. Some authors go as far as describing school mathematics as `...a rationality devoid of any content which can describe and therefore explain anything' (Walkerdine, 1988); or speaking of the `hidden curriculum' of mathematical education implanting a servile attitude towards technological questions into a large number of students (see Skovmose,1990).
In 1995, an inspiring `Call for Papers' was published by the International Committee on Mathematics Education (ICMI) under the title `Perspectives on the Teaching of Geometry for the 21st Century' (ICMI, 1995). This study asks the question: `Is there a crisis in the teaching of geometry?' The paper lists a number of evidences that can give cause to apprehension, such as omitting geometry from various secondary and tertiary mathematics curricula; or, the increasing gap between geometry as a modern science and as a school subject.
Another topic in the above-cited paper was the connection, or contrast, between the use of computers and the traditional methods of teaching geometry. In what ways should geometry curriculum be changed in countries which can afford the widespread use of computers, and in other countries which cannot?

And, finally, a question which is very near to the scope of the present paper: The `Modern Mathematics Movement' that got under way more than three decades ago, has led to the decline of the role of euclidean geometry in the curriculum. New attempts to restore euclidean geometry in its classical form have not proved very successful. What should we do? Would it be possible and advisable to include some elements of non-euclidean geometries into curricula?

The `Comparative Geometry' or `Plane-Sphere' project

If you come to see your project materialized after fifteen years of work, you probably tend to think that your project is the answer to all questions. I try to retain my good sense, and not to make claims like that. However, I do believe that my project can be used for addressing a number of interesting and important issues in mathematics education. I would consider it cheap and childish to list a number of problems, then triumphantly conclude the list with my project as the solution of all these problems. So I briefly relate to the essence of this project, in order to help the reader clearly understand my theoretical considerations and my biases.
The idea is to provide a collection of activities in comparative geometry between the plane and the sphere, for a wide range of ages and school types. The skills and techniques which are used in the activities are commonly used in plane geometry classes. One of the main ambitions was to make the material accessible to teachers who have experience in the usual middle and high school Euclidean plane geometry, but do not necessarily have any experience with any form of geometries different from Euclidean geometry.
It would have been impossible to teach this project without adequate manipulative devices. Therefore, it proved necessary to design construction tools in spherical geometry which correspond to the traditional drawing and measuring tools used to study geometry on the plane. These devices included: a thick sphere, about the size of a soccer ball; a tor us under the sphere for supporting it; hemispherical transparencies or `spherical draft papers'; compass; markers; and other accessories. In 1996, an American company, Key Curriculum Press, published my book: `Non-Euclidean Adventures on the Lenart Sphere'; and manufactured an educational kit, the Lenart Sphere Set (Lenart, 1996). The material has favourably been received by the American community of mathematics educators and teachers, as, for example, at the '96 Conference of National Council of Teachers of Mathematics, in San Diego, California.

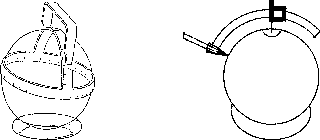
The concept of comparing plane geometry with spherical geometry in secondary or tertiary mathematics education has appeared in the works of authors as, for example, Henderson's `Experiencing Geometry on Plane and Sphere' (Henderson, 1995), or van den Brink's paper on `Spherical Geometry Lessons' (van den Brink,1994).

Axiomatic systems in the mathematics curriculum

Two main systems of axioms prevail in most curricula of mathematics. These systems are mostly present in an implicit form. Arithmetics and algebra are built on group and field theory, while geometry is based on Euclidean plane geometry. Any systems different from these two are usually treated as mathematical curiosities or historical asides. Very rarely are different systems studied with the same depth and rigor that is used in `standard' arithmetics, algebra, and geometry.
This limited interpretation suggests to the students that mathematics is a strictly hierarchical science. The message that students perceive can be characterized as follows: `Mathematics is built on a few ancient, never-changing, a priori systems which had been worked out by great scientists of the past. These systems can't and shouldn't be radically changed ever. Our teachers' task is to interpret the revelations of those great scientists for us. On our own, we are only capable of solving exercises of minor importance, dealing with insignificant details of the grand theories.'
The question is: Is this approach good or bad? Shall we consider it an advantage or disadvantage that the curriculum focuses on a few, relatively closed and safe systems of mathematics?
Personally, I feel that this approach is very far from what I call `mathematics'. For me, modern mathematics is about the freedom of choice. One is free to choose any system of axioms to solve a given problem. He/she is responsible for this choice in the sense that the way of solving the problem has to prove the consistency and usefulness of the chosen system of axioms. However, I am aware that I have spent much more time on mathematics than a lay person, and my personal taste does not necessarily coincide with the needs of the whole community. Are there some reasons of more objective character to decide between the choices `One system for one mathematical discipline' versus `Alternative systems for one and the same mathematical discipline'?

What does the society and the individual expect from the mathematics curriculum?

The curriculum of school mathematics reflects the needs of the society and the individual, regarding the ways and means of socializing the individual. This can clearly be seen in countries which have had to survive a number of changes in the social, economical, and political system. For example, Hungary has gone through at least six fundamental changes in the last one hundred years, with all the due consequences in the educational system. In this connection, it is very illuminative to read Taylor's analysis on the interdependence of politics and education, with a special stress on South African problems (Taylor, 1991).
What does society expect from education? The two extremes can be summed up in the question: Does society require of the school system to produce obedient subjects in a hierarchical system, or, rather, citizens of independent mind and thinking, capable of understanding their own situation and chances, and also of their fellow citizens?
As I mentioned above, I look upon mathematics as a science about the freedom of choice. This means that modern mathematics creates a multitude of decision situations, `points of decision' (Streefland, 1992). In this manner, modern mathematics, if properly taught, encourages and develops students' ability to prepare themselves for decision making situations, and to make their own decisions. The value of this ability within the school depends on its value in `real' life. If an average citizen has a number of opportunities in his/her life to make decisions in situations of great consequence, then it is well expectable from education to help him/her develop this ability. However, if the financial, economical, or political constraints deprive the individual of making important decisions even in his or her personal life whether the small minority of real decision makers are called `mister', `comrade', or `professor', then the ability of decision making may prove not only superfluous, but explicitly harmful, leading to deep, even fatal, conflicts between the individual and the society.
What does the individual expect from the mathematics curriculum? Again, this expectation depends on the individual's chances in life. A person who cannot exert a real influence on his/her world or society, will find a hierarchical mathematical theory, based on one fixed system of axioms, much closer to real life situations than a comparative system of alternative models. On the other hand, an individual who has grown accustomed to making important decisions in the private and/or public life will find it degrading, even boring, to be reduced to trifling matters in mathematics.
This tendency is strengthened by the effects of information revolution. A member of a modern democratic society, who has access to a wide range of economical, political, scientific, educational, etc. information, is very likely to refuse the role of a passive recipient in an educational process. In order to survive and succeed, mathematics education has to create curricula `where pupils are expected to take an active part in the development of their mathematical knowledge' (ICMI, 1995).
The individual also needs that the mathematics curriculum provides `real' real-world problems, not `dressed-up' problems where the role of the context is nothing more than cosmetics (De Lange, 1994).

The computer in the geometry curriculum

Computers have become an indispensable part of our everyday life and environment, including our schools. I believe that computers are just as important and helpful in teaching geometry as in any other branch of mathematics. For example, many details of the Plane-Sphere project can very well be demonstrated on educational software. Even a hand held calculator proves very handy when the student has to perform tedious trigonometric computations in plane or spherical geometry.
I would like to caution against one thing. It seems aimless to develop a kind of mathematical snobbery in the children about the use of the computer as the exclusive way of performing geometric experiments. I think that a wide variety of experimental techniques should be offered for the students, the computer being one, if very important, among other methods. If the process of actually drawing a line on the plane or on the sphere becomes an elementary action for the student (see Lenart, 1993), then we can safely proceed to drawing the line on the computer screen. However, in my opinion, the step of drawing manually on various surfaces cannot be left out from the learning process.

Non-euclidean geometries

In the above-mentioned ICMI paper, I found: `Aims:... To supply an example of an axiomatic theory.' I think that a single example of an axiomatic theory has no real educational value. In order to properly teach axiomatization, we need at least two different systems of axioms on the same subject. It is necessary that these two systems represent the same, or nearly the same, degree of difficulty. Hyperbolic geometry, that is, the Bolyai-Lobachevsky geometry, is too difficult to compare with the well-known Euclidean plane geometry. Alexandrov remarks: `Lobachevskian geometry can hardly be included in secondary school curricula, but it seems essential to give pupils and idea of it, and to show them the greatness of the human spirit...' (Alexandrov, 1994). My own experiences show that comparing spherical geometry with plane geometry makes it possible to introduce some concepts and theorems of hyperbolic geometry in a natural and understandable manner. In this way, some basic elements of this geometry can be used and modellized in the secondary curriculum (see, for example, Szilassy, 1995).

Short conclusion and proposal

As I mentioned before, I think that several of the above-mentioned problems in the teaching of geometry can be influenced by introducing comparative geometry in secondary education. I do not even dream of establishing comparative geometry as the only form of geometry teaching; but I believe that this project deserves to be one of the 12-15 basic topics on which an adequate mathematics curriculum in the secondary school can be built. I add that various alternative models could be introduced of advantage in several other parts of the mathematics curriculum, as, for example, in algebra, topology, or number theory.

References

Alexandrov, A. (1994). Geometry as an element of culture. In: Proc. of ICME-7. Vol. 1. 365-368.

Balacheff, N. (1990). Future perspectives of research in the psychology of mathematics education. In: Mathematics and Cognition. ICMI Study Series. Cambridge University Press, 135-148.

Brink, Jan van den (1994). Spherical geometry lessons. Mathematics Teaching, 147 (June 1994), 26-33.

Cooney, T. (1994). The Public image of mathematics and mathematicians. In: Proc. of ICME-7, Vol. 2., 219-225.

Henderson, D. (1995). Experiencing geometry on plane and sphere. Prentice Hall, Englewood Cliffs, New Jersey.

ICMI (The International Commission on Mathematical Instruction) (1995). Perspectives on the teaching of geometry for the 21st century. Educational Studies in Mathematics, 28, 91-98.

Lange, Jan de (1994). Curriculum change: an American-Dutch perspective. In: Proc. of ICME-7. Vol. 1. 229-248.

Leder, G. (1980). Bright girls, mathematics and fear of success. Educational Studies in Mathematics, 11, 411-422.

Lenart, I. (1993). Alternative models on the drawing ball. Educational Studies in Mathematics, 24, 277-312.

Lenart, I. (1996). Non-Euclidean adventures on the Lenart Sphere. Key Curriculum Press, Berkeley.

Skovmose, O. (1990). Mathematical education and democracy. Educational Studies in Mathematics, 21, 109-128.

Streefland, L. (1992). Letter to the author. February 11, 1992.

Szilassy, l. (1995). The Bolyai. Exe Software (Constructions in Hyperbolic Geometry). In Hungarian.

Taylor, N. (1991). Independence and interdependence: analytical vectors for defining the mathematics curriculum of schools in a democratic society. Educational Studies in Mathematics, 22, 107-123.

Walkerdine, V. (1988). The mastery of reason. Routledge, London.

ICME8 - WG13 - 02 JUL 96

[Next] [Previous] [Top]

Generated with CERN WebMaker

From geometry to geometries: an aspect of changing the geometry curriculum